Kepler Second Law

\documentclass[border=2pt]{standalone}

% Drawing
\usepackage{tikz}

% Notation
\usepackage{physics, bm}

% Tikz Library
\usetikzlibrary{angles, quotes}

% Newcommand
%% Polar Coordinates 
\newcommand{\pcord}[3]{\coordinate (#3) at ({#1*cos(#2)},{#1*sin(#2)});}

\begin{document}
	
	% Macros
	\def\r{2.22}
	\def\rr{3.22}
	
	\begin{tikzpicture}[line cap = round]
		% Grid
%		\draw[thin, dotted] (0,0) grid (8,8);
%		\foreach \i in {1,...,8}
%		{
%			\node at (\i,-2ex) {\i};	
%		}
%		\foreach \i in {1,...,8}
%		{
%			\node at (-2ex,\i) {\i};	
%		}
%		\node at (-2ex,-2ex) {0};
		
		% Coordinates
		\coordinate (O) at (0,0);
		\pcord{\rr}{12.5}{A}
		\pcord{\r}{12.5}{B}
		\pcord{\rr}{-14.5}{C}
		\pcord{\r}{-14.5}{D}
		\pcord{1.54}{-14.5}{E}
		\coordinate (F) at (\r,0);
		% Nodes
%		\node at (A) {A};
%		\node at (B) {B};
%		\node at (C) {C};
%		\node at (D) {D};		
%		\node at (E) {E};
%		\node at (F) {F};	
		
		% Orbit
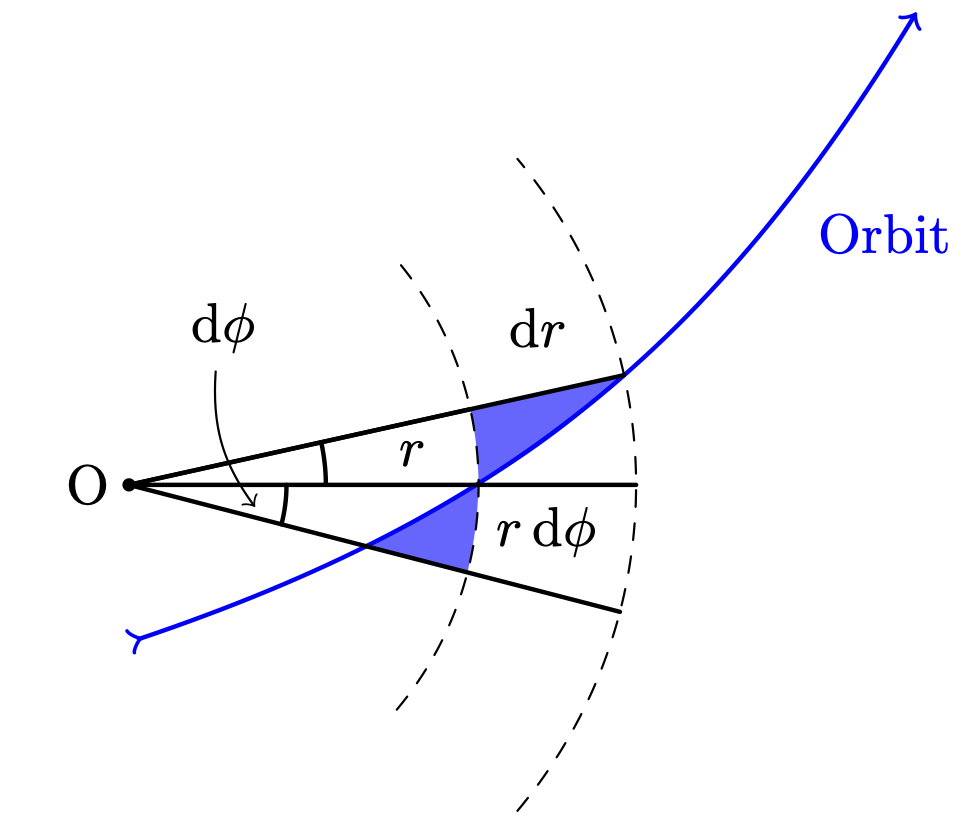
		\draw[shift={(0,-1)}, thick, >->, blue] (0,0) to [bend right = 20] (5,4) node [shift={(-0.2,-1.4)}] {Orbit};
		
		% Nodes
		\node at (2.6,1) {$\dd{r}$};
		\node at (1.8,0.2) {$r$};
		\node at (2.65,-0.3) {$r\dd{\phi}$};
		\node (a) at (0.6,1) {$\dd\phi$};
		
		% Paths
		\path[fill=blue, opacity=0.6] (F) to[bend right = 5] (B) -- (A) to[bend left = 6] cycle;
		\path[fill=blue, opacity=0.6] (F) to[bend left = 5] (D) -- (E) to[bend right = 4] cycle;
		
		% Circles
		\draw[domain=-40:40, dashed, variable=\t, samples=100] plot ({\r*cos(\t)},{\r*sin(\t)});
		\draw[domain=-40:40, dashed, variable=\t, samples=100] plot ({\rr*cos(\t)},{\rr*sin(\t)});
		
		% Lines
		\draw[thick] (O) -- (\rr,0);
		\draw[thick] (O) -- (A);
		\draw[thick] (O) -- (B);
		\draw[thick] (O) -- (C);
		\draw[->] (a) to[bend right=20] (0.8,-0.14);
		
		% Point
		\draw[fill=black] (0,0) circle [radius=1pt] node [left] {$\mathrm{O}$};
		
		% Angles
		\pic[draw, thick, angle radius = 1cm] {angle = E--O--F};
		\pic[draw, thick, angle radius = 1.25cm] {angle = F--O--B};
	\end{tikzpicture}
	
\end{document}

Kepler_Second_Law.tex