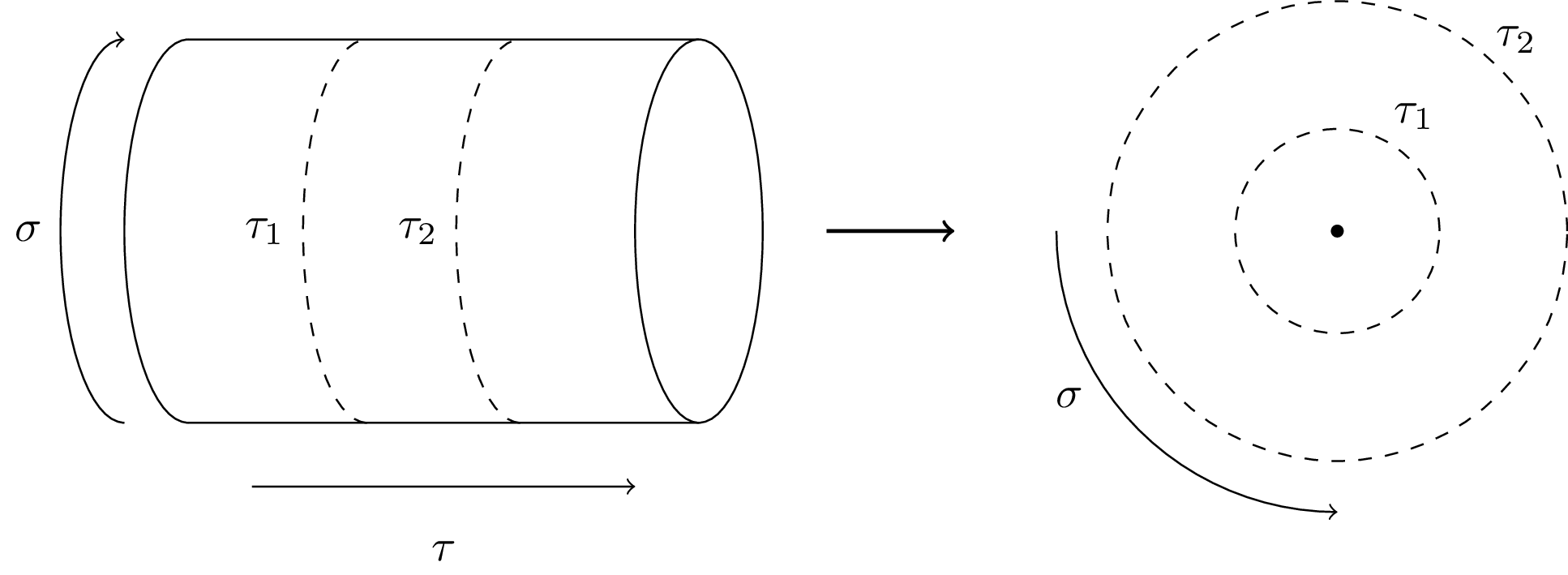
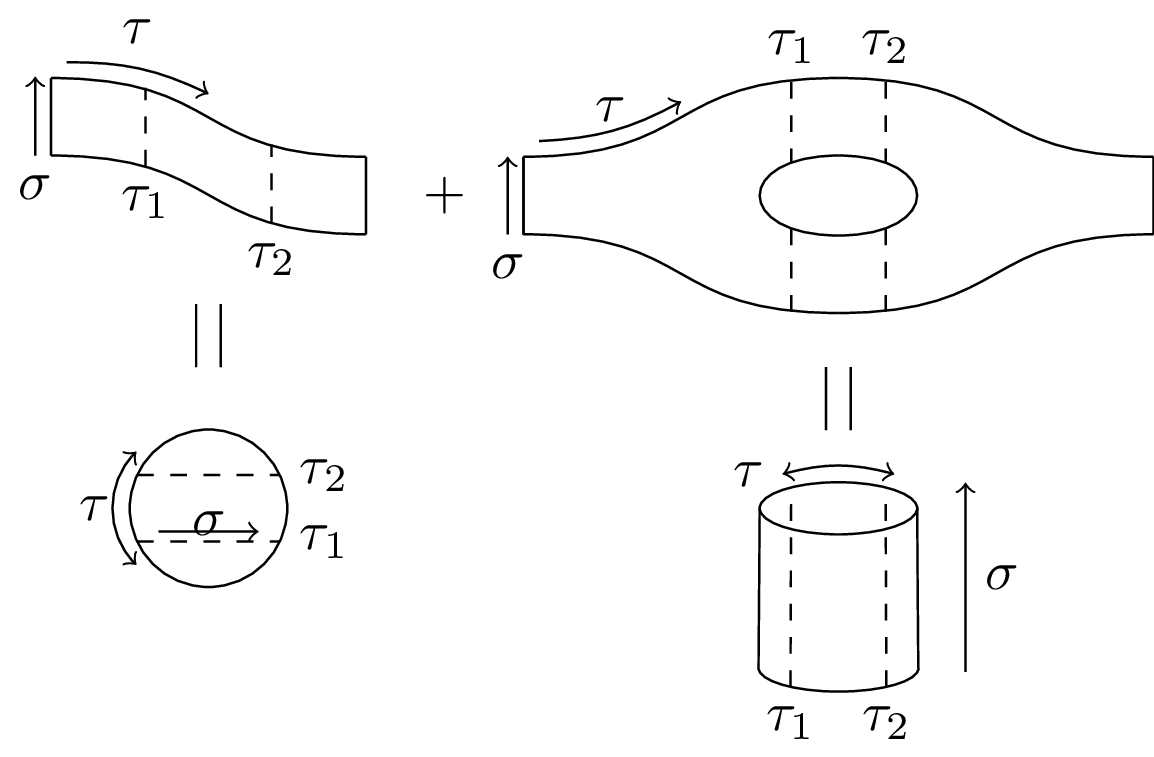
String theory: Primary fields and radial quantization A time-ordered product of fields on the cylinder maps to a radially ordered product in the complex plane. This graphic visualizes how different times on the cylinder correspond to different times on the plane.
Edit and compile if you like:
% String theory: Primary fields and radial quantization% A time-ordered product of fields on the cylinder maps to a radially ordered product in the complex plane.% This graphic visualizes how different times on the cylinder correspond to different times on the plane.\documentclass[tikz]{standalone}\begin{document}\begin{tikzpicture}\draw[dashed] (2.6,0) arc (-90:90:-0.5 and 1.5);% line 1\draw[dashed] (1.4,0) arc (-90:90:-0.5 and 1.5);% line 2\draw[->] (-0.5,0) arc (-90:90:-0.5 and 1.5);\draw (0,0) -- (4,0);% bottom line\draw (0,3) -- (4,3);% top line\draw (0,0) arc (270:90:0.5 and 1.5);% left half of the left ellipse\draw (4,1.5) ellipse (0.5 and 1.5);% right ellipse\draw (0.6,1.5) node {$\tau_1$};\draw (1.8,1.5) node {$\tau_2$};\draw (-1.25,1.5) node {$\sigma$};\draw[->] (0.5,-0.5) -- (3.5,-0.5);\draw (2,-1) node {$\tau$};\draw[->,thick] (5.0,1.5) -- (6,1.5);\draw[dashed] (9,1.5) circle (0.8);\draw[dashed] (9,1.5) circle (1.8);\draw [->,domain=0:90] plot ({9 - 2.2*cos(\x)},{1.5-2.2*sin(\x)});\draw (6.9,0.2) node {$\sigma$};\draw (9.6,2.4) node {$\tau_1$};\draw (10.4,3) node {$\tau_2$};\node at (9,1.5) [circle,inner sep=1pt,fill=black] {};\end{tikzpicture}\end{document}
Click to download: cylinder-to-plane.tex
Open in Overleaf: cylinder-to-plane.tex
This file is available on tikz.netlify.app and on GitHub and is MIT licensed.
See more on the author page of Janosh Riebesell..